7 Rich problems as building blocks for mathematics education

Student teachers are often confronted during their education with rich mathematical problems. Such rich problems both invite a diversity of mathematical activities and reveal sections of the instructional theory. Rich problems demonstrate that mathematics can be widely applied. Student teachers are amazed by, for instance, what they can do with their own mathematical knowledge and background. Solving rich problems encourages them to work together and stimulates the development of a mathematical disposition. Rich problems also play an important role in primary education. They act as beacons in subject matter domains and provide the opportunity to visualize, schematize and model reality. They stimulate interaction and collaboration by students in primary school. They reveal to children the applicability of mathematics. The point of departure for the primary school instructional theory is created by students’ experiences with rich problems. Reflection on these experiences offers students the opportunity to work with rich problems themselves with the children at the primary schools (Goffree, 1979).

Walking to Marseille

On a map of Europe (scale: 1: 15,000,000), the distance from Amsterdam to Marseille is 7 cm. How many kilometers is this distance in reality? How can you turn this into a rich mathematical problem? Idiscuss this matter of instructional theory with my students. They do not need much time to think my questions over. Almost immediately, one cries out, "you have to situate it within the children’s experiences. "Another continues, "a vacation in Marseille." I write on the board: ‘you’re going on vacation to Marseille’. We’re on our way. I ask the students whether they can think of any more ways to enrich this word problem. One of them suggests the children’s own contributions: have them look up the distance between Amsterdam and Marseille in an atlas or on a road map. Another student follows a different train of thought: "you can have the children figure out how long it will take you to get there, or how much gas you’ll need."

My response to these suggestions is that, although this would indeed enrich the situation, we were actually searching for an enrichment of the problem that would maintain the original mathematical situation - which was about distance. Fred has a bright idea: "what if I tell the children that I’m going to hike to Marseille? I have three weeks vacation. Will I make it?" Not all the students are directly aware of the beauty of Fred’s suggestion. I ask Fred to explain it in more detail. Fred knows exactly what he means, and replies, "this way you get the children to concentrate on the distance between Amsterdam and Marseille." I had written a list of characteristics of a good text problem on the back of the board, as that was what we were busy doing.

What characterizes ‘rich problems’?

  • solving rich problems generally leads to mathematical activities;
  • different approaches are permitted for solving rich problems;
  • rich problems are formalized in recognizable situations, which are often taken from everyday life;
  • rich problems are by nature open-ended;
  • rich problems often share common ground with other disciplines;
  • rich problems appear in a great variety of forms: puzzle, brief text, story, newspaper clipping, et cetera.

    •  

     
     
     
     
     

    Why are rich problems so important?

  • rich problems offer various possibilities for mathematization and didactization (Freudenthal, 1991);
  • rich problems invite the students to enter into mathematics and its mathematics education;
  • rich problems provide opportunities to cross borders, both in a mathematical and a theoretical sense;
  • rich problems provide opportunities for visualization, modeling and schematization;
  • rich problems expose the applicability of the mathematics;
  • solving rich problems contributes to the development of a mathematical disposition;
  • rich problems encourage collaboration and interaction;
  • rich problems reveal the connection between different subject matter domains.

    •  

     
     
     
     
     

    How can rich problems be introduced?

  • rich problems can be introduced at the institute as a way of accessing subject matter components;
  • rich problems can provide a foothold for long-term learning processes;
  • rich problems can play a role in acquiring numeracy
  • rich problems can be introduced to help students learn to reflect on their mathematical disposition;
  • rich problems are excellent examples for use during internships;
  • rich problems can be used to elicit reflection on important theoretical principles.

    •  

     
     
     
     
     
     
     

    Getting down to work at the institute for teacher education

    Rich problems play a crucial role throughout the course of study. Helping the students to develop a feeling for rich problems can serve as a significant aid in structuring the education. Students must develop a good eye for rich mathematical problems. And they - with their knowledge of so many subjects - may have the advantage here. It is the educator’s responsibility to situate rich problems in a theoretical perspective. The following are a number of points for attention:

  • colleagues with a background in a different discipline can be a source for rich problems. Take, for instance, biology, health and hygiene, geography, visual arts, physical education, history, music, and languages;
  • special students can be a source of rich problems, thanks to their specific backgrounds;
  • students can be sent to search for and investigate rich problems;
  • the educator in mathematics education has a broad range of interests; (s)he reads newspaper and magazine articles to develop a good eye for rich problems;
  • now and again, an educator seizes a discussion taken from the internship practice for educational enrichment.

    •  

     
     
     
     
     
     
     

    8 Constructive analysis

    Constructive analysis is, in the first place, analysis. It entails analysing subject matter for primary school mathematics, which is subject matter that has been more or less theoretically processed. Why should primary school teachers need to analyze this? On the whole, they do not; perusal of new materials on the educational market often remains limited to superficial browsing, which leaves one with a subjectively tinted impression. It is quite a different matter, however, if one is planning to acquire new material for actual use in the classroom. In that case, the material is examined more meticulously, and an attempt is perhaps also made to imagine how it could be applied and how certain children would respond to it. Then, the material is analyzed from the perspective of ‘to buy or not to buy’. The situation shifts yet again if the teacher is being obliged to use material in the classroom that is new (to him or her). Substitute teachers are often faced with this, as are teachers who are teaching a given grade for the first time. But even a teacher’s decision to take interesting worksheets or suggestions for lessons from a familiar source requires more than a superficial perusal of the material. If the situation described here occurs with a mathematics textbook, then the teacher’s guide will generally relieve the teacher of much of the preparatory work. At the same time, however, a (detailed) teacher’s guide takes personal contributions and emphasis out of the teacher’s hands. At the very least, this creates a situation in which a teacher, in a docile and subservient fashion, finds herself encouraging the students to take initiative, perform activities and engage in reflection. Is a contradiction in terms perhaps concealed here?

    We now come to constructive analysis. The adjective ‘constructive’ indicates the teacher’s active and reflective contribution to the analysis of material for classroom use. This teacher will both take the initiative and make grateful use of the ideas and elaborations of others. Regarded from this perspective, constructive analysis is a form of educational design and is part of one of the lines of development that lead to professionalization. Where primary school mathematics is concerned, constructive analysis forms a significant component of teacher education. If mathematics education is to remain ‘realistic’, one cannot restrict oneself year after year to what was included (long ago) in the textbooks.

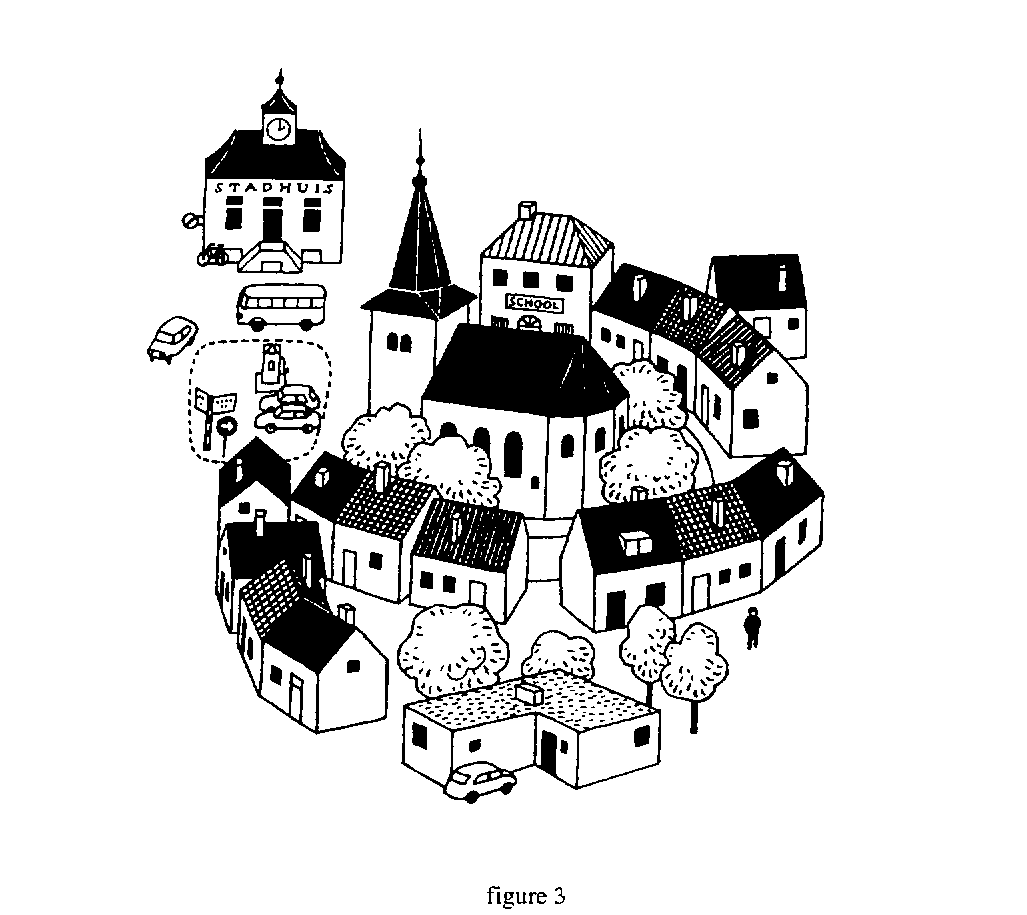
    Aerial view of a village: an example

    We now turn to page 174 of the third edition of Wiskunde & Didactiek4(Goffree, 1989), (fig.3). This page shows a worksheet with an illustration of an aerial view of a village. The accompanying text states: ‘The teacher’s instructions for this worksheet are as follows: this is a village, seen from above. In fact, it is a kind of aerial view of a model made out of matchboxes.’ The investigation concentrates on this village. The question is whether it can determined with any certainty how many people live here. This question may be posed indirectly by, for instance, asking the size of the primary school that is just visible behind the large church tower. The children’s learning activities involve such things as systematic counting, the concept of ‘average’, family size, the (intuitive) idea of random sampling, population composition, and simple operations. The circumstances are important: only approximate calculation is possible here and more than one answer may well be correct, each supported by sound reasoning. The objective of this bit of education is not, therefore, to find one (unequivocal) answer. It goes farther: the objective is to find investigative activities, within which the above-mentioned concepts acquire real meaning, and through which students can gain the opportunity to sustain their own convincing arguments and also appreciate those of others.

    Furthermore, the students are guided through a kind of thought experiment, in which problems are spotted, formulated and solved, and brought to a conclusion. In the meantime, attention is devoted now and again to what primary school children might be able to do with this problem situation.

    Steps

    Wiskunde & Didactiek recommends this problem situation as a good starting point for a mathematics education project. The following steps are suggested in undertaking such a project:
    1 First solve the problem yourself.
    2. Pose yourself questions and find the answers.
    3 Describe reflectively your own solution process.
    4 Where possible, look across the borders of the mathematical subject matter.
    5. Adopt a theoretical disposition and try to imagine how your students would react.
    6. Important: how do you intend to introduce this problem situation? Make up introductory problems.
    7. Think about which question you will pose first.
    8. How do you think the children will respond to this?
    9. Think up essential questions you can use to follow up; these provide structure to the education.
    10. Difficult moments will certainly occur, which require explanation or some organization. How will you deal with this?
    11. Have you reserved something special for particular sections?
    12. The children must also be allowed time to think things over (reflective moments). Et cetera.

    Student difficulties

    Experiences with constructive analysis have shown that students do not find this easy. Their difficulties are perhaps a signal to the educator that this topic should be presented in sections.

  • Students have difficulty choosing a topic on their own.
  • Students usually think too quickly in terms of subject matter and educational theory, whereas they should first spend time becoming acquainted with the possibilities and the difficulties of the topic.
  • Often only afterwards do students see the point of beginning on one’s own level and solving all the problems and assignments oneself.
  • Students must gradually acquire a sense of which material lends itself to the approach of constructive analysis.
  • Students must regard this manner of lesson preparation as a component in their entire teacher education, and understand that the development of increasing independence self-sufficiency) is included in this.
  • Particularly difficult - but proven to be extremely motivating - is the search for material in one’s own environment (hobby, sport, part-time job, parents’ occupation, et cetera).

    •  

     
     
     
     
     

    Appropriate assignments

    A search is therefore made for assignments which allow for constructive analysis. The earlier example of the aerial view of the village belongs to the category of ‘rich problems’, of which every educator has a favorite ‘top ten’. For example for Dutch educators: the surface area of the Netherlands, Van Gogh, a billion seconds, the towers of Hanoi, grains of corn on a chess board, 18,000 babies, Szygmund, on the road to Paris, Egbert the giant, the Hans-problem. Less open-ended than these, and placing fewer demands on individual creativity and contributions are, for instance: a student worksheet, a project taken from the primary school textbook De wereld in getallen5, a geometrical (visual) problem taken from the primary school textbook Rekenen & Wiskunde5, or from Rekenwerk5. Students who use a journal well, will now and then note constructive analyses of bits of subject matter that come their way, for instance, while browsing through a mathematics textbook, reading the newspaper, or watching a children’s television program.

    Notes

    1 Dutch acronym for National Association for the Development of Mathematics Education.

    2 The following subjects always appear in the primary school curriculum in the Netherlands, if possible in an integrated form:

  • sensory and physical education
  • Dutch
  • arithmetic and mathematics
  • English
  • a number of factual subjects, including geography, history, science (biology), social structures (including civics) and religious movements
  • creative activities, including the use of language, drawing, music, handicrafts, play and movement
  • self-reliance, i.e. social and life skills, including road safety
  • health instruction

    • Schools in the province of Friesland must also teach Frisian and may conduct some lessons in that language. In the case of children with a non-Dutch background, some lessons may likewise be conducted in their own native language.
    English is taught to the top two classes in primary schools.
    Curriculum content and teaching methods are not prescribed.
    There is, however, a National Institute for Curriculum Development (SLO) with responsibility for developing curricula and models or alternative models for school work and sections of work plans. The schools can make use of these if they wish.

    3 Dutch institute for primary teacher education.

    4 Wiskunde & Didactiek is a text book used at Dutch primary school teacher education institutes.

    5 De wereld in getallen, Rekenen & Wiskunde and Rekenwerk are primary school text book series.

    References

    Brink, F.J. van der (1 989). Realistisch rekenonderwijs aan ionge kinderen. Utrecht: OW&OC.

    Buys, K. (1991). Telactiviteiten voor kleuters. Baarn: Bekadidact.

    Freudenthal, H. (1975). Wandelingen met Bastiaan. In: Feestboek Prof. dr. Hans Freudenthal - 70jaar.

    Freudenthal, H. (1991). Revisiting Mathematics Education. China lectures. Dordrecht: Kluwer.

    Galen, F. van, M. Dolk, E. Feijs, V. Jonker, N. Ruesink, W. Uittenbogaard (1989). Interactieve video in de nascholing rekenen-wiskunde. Utrecht: CD-b.

    Gipe & Richards, (1992). Reflective thinking and growth in novices' teaching abilities. Journal of Education Research, vol. 86, nr.1

    Goffree, F.(1979). Leren onderwijzen met wiskobas. Onderwijsontwikkelingsonderzoek 'wiskunde en didaklie' op de pedagogische akademie. Utrecht: IOWO.

    Goffree, F. (1985). Wiskunde & didactiek deel 3. Groningen: Wolters Noordhoff.

    Goffree, F. (1992). Wiskunde & didactiek deel 2. Groningen: Wolters Noordhoff.

    Goffree, F. (1993). Kleuterwiskunde. Groningen: Wolters Noordhoff

    Goffree, F. (1994). Wiskunde & didactiek deel 1. Groningen: Wolters Noordhoff.

    Goffree, F. & M. Dolk (1995). Proeve van een nationaal programma rekenen-wiskunde & didactiek op de pabo. Enschede/Utrecht: SLO/NVORWO.

    Gudmundsdottir, S. (1991). Story-maker, story-teller: Narrative structures in curriculum. Journal of Curriculum Studies. Vol. 23, no.3,  207-218.

    Harvey, R., D. Kerslake, H. Shuard & M. Torbe (1982). Language Teaching and Leraning. No.6, Mathematics. London: Ward Lock Educational.

    Holt, J. (1965). How children fail. New York: Dell.

    Inspectie van het Onderwijs (1989). Overwegingen. Rapportage van een proefvisitatie. De Meern.

    Moust, J.H.C.M., P.A.J. Bouhuijs & H.G. Schmidt (1989). Probleemgestuurd leren. Groningen: Wolters Noordhoff.

    Nijhof, W.J., H.A.M. Franssen, W.Th.J.G. Hoeben & R.G.M. Wolbert (1993). Handboek Curriculum. Modellen, theorieën, technologieën. Amsterdam: Zwets & Zeitlinger.

    Puik (1992). Verhalen van de lerarenopleiding. Op zoek naar kwaliteit van opleidingsonderwijs. Noordwijkerhout: SLO-NVORWO.

    Puik (1993). Uitlijnen. Op zoek naar kwaliteit van opleidingsonderwijs. Enschede: SLO/NVORWO.

    Puik (1994). Standaards voor rekenen-wiskunde & didactiek. Een proeve. Enschede: SLO.

    Schön, D. (1983). The Reflective Practitioner. New York: Basic Books.

    SLO (1982). Modelonderwijsleerplan PABO. Enschede: SLO

    Streefland, L. (1988). Realistisch breukenonderwijs: onderzoek en ontwikkeling van een nieuwe leergang. Utrecht: OW&OC.

    Treffers, A.J., E. de Moor & E. Feijs (1989). Proeve van een nationaal programma voor het reken-wiskundeondervijs op de basisschool. Deel 1. Overzicht einddoelen. Tilburg: Zwijsen.

    Wijdeveld, E.J. (1991). Veldaanvraag bij de SLO. Utrecht: NVORWO.

    Appendix

    Table of contents of the Dutch publication Proeve van een nationaalprogramma rekenen- wiskunde & didactiek op de pabo.

    The numbers between brackets refer to the chapter in this publication.

    Foreword

    Introduction (1)

    Part 1: The teaching profession (background)

    The foundation of mathematics education

    1.1 Student numeracy

    1.2 Mathematics education in practice

    1.3 The theory

    1.4 Reflection (3)

    1.5 The program

    1.6 In perspective: profile of an ideal mathematics teacher

    1.7 The learning environment at the institute for primary teacher education

    1.8 Professional growth

    1.9 Gauging, weighing and evaluating students

    1.10 Signs of realistic mathematics education

    Standards for mathematics education at institutes for primary teacher education (2)

    2.1 Introduction

    2.2 The standards as spotlight

    2.3 Eighteen standards for mathematics education

    2.4 The repertoire of the mathematics educator

    Part 2: In the classroom

    Presenting knowledge

    3.1 Introduction

    3.2 Narratives

    3.3 Lectures

    3.4 Mathematics study groups

    3.5 Self-study

    3.6 A straightforward mathematics class

    3.7 The introductory course for the subject area of mathematics education

    3.8 Theoretical reflections (4)

    3.9 Presenting paradigms (5)

    Coaching students

    4.1 Introduction

    4.2 Coaching in the domain of numeracy

    4.3 Coaching in the area of actual classroom practice

    4.4 Coaching self-study

    4.5 Coaching towards graduation

    4.6 Coaching the making of a journal

    Collaboration for actual classroom practice

    5.1 Introduction

    5.2 Preparing a lesson together

    5.3 Collaborating on organizing the internship as a leamin. environment

    5.4 Drawing up an educational contract together

    5.5 Practical experience at the institute for primary teacher education

    5.6 Referring to other sources

    5.7 Discussion after the fact after the lesson

    5.8 Consulting the counselor together

    5.9 Taking reflective notes

    5.10 Designin. education to-ether with other students

    Gauging the professionalization

    6.1 Introduction

    6.2 Questions on different levels

    6.3 Evaluating and inventing written exam problems

    6.4 Preparing and assessing oral exam

    6.5 The educational dossier: viewing the level of professionalism

    6.6 Evaluating projects

    6.7 Discovering students’ viewpoints

    Designing education

    7.1 Introduction (6)

    7.2 Openings

    7.3 An educational tour de force

    7.4 Rich problems as building blocks for mathematics education (7)

    7.5 Designing a module

    7.6 Designing materials for self-study

    7.7 Constructive analysis (8)

    7.8 Gems of mathematics education design

    7.9 Designing for kindergartners

    7.10 Contributing to problem-guided instruction

    7.11 The educational workplace

    Determining the educational course load offered

    8.1 Introduction

    8.2 Primary school

    8.3 The core program at the institute for primary teacher education

    8.4 The younger child

    8.5 Specializing in the older child

    8.6 Diagnosing and diagnostic education

    8.7 An educational arrangement: problem-guided education (PGO)

    8.8 The computer in mathematics education

    Part 3: The output

    Requirement for proficiency in teaching mathematics

    9.1 Introduction

    9.2 The subject mathematics at the primary school

    9.3 The teacher teaching mathematics

    9.4 Requirement for proficiency in teaching mathematics at the primary school