|
 |
| |
| Abstracts |
| |
|
N. Chemetov (Lisbon, Portugal)
The motion of the rigid
body in a viscous fluid with collisions |
| We consider the problem of the
motion of a rigid body in an incrompressible viscous fluid, filling a
bounded domain. This problem was studied by many authors. They
considered classical non-slip boundary conditions, which gave them very
PARADOXICAL result of no collisions of the body with the boundary of the
domain. In this work we
study when Navier slip conditions are prescribed on the boundary of the
body (instead of non-slip conditions). We prove for this model the
GLOBAL existence of weak solution, which permit COLLISIONS with the
boundary of the domain.
|
|
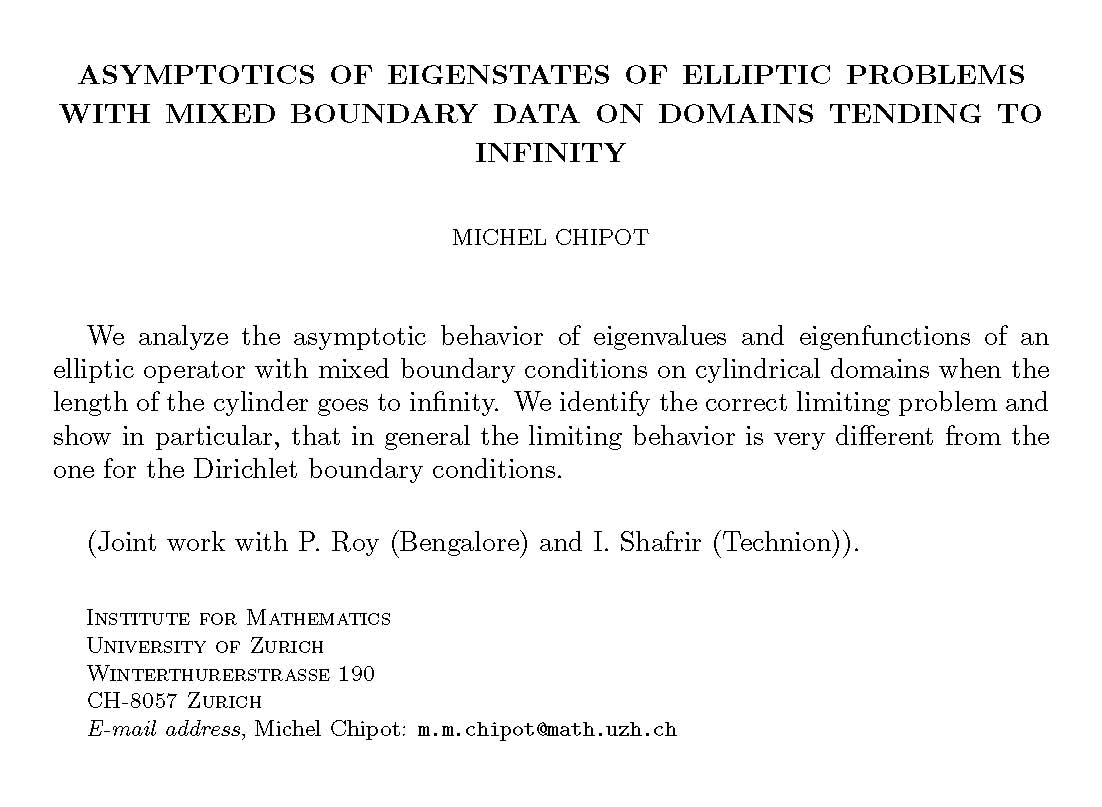
M. Chipot (Zurich, Switzerland)
Asymptotics of eigenstates
of elliptic problems with mixed boundary data on domains tending to
infinity |
 |
|
J.I. Díaz (Madrid, Spain)
On the free boundary for
quenching type parabolic problems via local energy methods |
| In his pioneering paper, Some problems with
free boundaries for the degenerating equations of gas dynamics. (Dinamika
Sploshnoi Sredy, Novosibirsk, 1973, Vyp. 13, pp. 5-17. (Russian))
Stanislav Antontsev proposed the idea of a general method to prove the
existence and location of free boundaries for quasilinear parabolic
equations of degenerate type. Since then, many other paper developed
this clever idea giving rise to a general methodology which today is
applicable to nonlinear partial differential equations on any type (elliptic,
parabolic and hyperbolic) and of any order (higher order too) and
systems (see, e.g. the monograph: S.N. Antontsev, J.I, Diaz and S.I.
Shmarev, Energy Methods for Free Boundary Problems: Applications to
Nonlinear PDEs and Fluid Mechanics, Birkhäuser, Boston, 2001.
The main goal of this lecture is to present the
application of this kind of energy methods to the case of equations
involving terms with negative exponents (the so called quenching
problems).
|
|
G. Galiano (Oviedo, Spain)
Applying Energy |
| We show how the Energy Method is used to deduce
the existence of free boundaries and some of their properties in two
problems expressed in terms of systems of PDE's. One is a classical
problem in fluid dynamics, the Boussinesq-Oberbeck system. The other
comes from the modeling of soil salinization. |
|
S.L. Gavrilyuk (Marseille, France)
Hyperbolic model of
hyperelasticity and applications to high impact problems |
| We consider the equations of hyperelasticity
for isotropic solids in the Eulerian coordinates in a special case where
the specific stored energy is a sum of two functions. The first one, the
hydrodynamic part of the energy, depends only on the solid density and
the entropy. The second one, the shear energy, depends on the invariants
of the Finger tensor in such a way that it is unaffected by the volume
change. A new sufficient criterion of hyperbolicity for such a system
containing 14 unknowns is formulated : if the hydrodynamic sound
velocity is real and a symmetric 3x3 matrix defined in terms of the
shear energy is positive definite on a one-parameter family of surfaces
of the unit-determinant deformation gradient, the equations are
hyperbolic. A thermodynamically consistent
multi-phase extension of the hyperelasticity is proposed for solving
fluid – structure interaction problems with irreversible elasto-plastic
transformations. Some applications are presented : fracture formation
and spallation under high velocity impact.
|
|
S. Kamin (Tel Aviv, Israel)
Prescribed conditions at
infinity for parabolic equations |
 |
|
A. Meirmanov (Almaty, Kazakhstan) and S.
Mukhambetzhanov (Almaty, Kazakhstan)
Mathematical models of a
wormhole formation |
| In the present talk we consider a free boundary
problem describing the wormhole formation in the porous media at the
microscopic level. The mathematical model consists of the Stokes system
for the velocity and pressure of the liquid and the diffusion-convection
equation for the concentration of the acid. Using the two-scale
convergent method for non-periodic structures we derive homogenized
system with unknown coefficients depending on the structure of the pore
space. In turn, this structure is defined by the unknown concentration
of the acid. |
|
H. de Oliveira (Algarve, Portugal)
Analysis of the existence
for the steady Navier-Stokes equations with anisotropic diffusion (joint
work with S.N. Antontsev) |
| The boundary-value problem for the generalized
Navier-Stokes equations with anisotropic diffusion is considered in this
talk. For this problem we prove the existence of weak solutions in the
sense that solutions and test functions are considered in the same
admissible function space. We prove also the existence of very weak
solutions, i.e., solutions for which the test functions have more
regularity. By exploiting several examples we show, in the case of
dimension 3, that these existence results improve its isotropic versions
in almost all directions or for particular choices of all the diffusion
coefficients. |
|
V. Shelukhin (Novosibirsk, Russia)
Homogenization of time
harmonic Maxwell equations and the frequency dispersion effect |
| We perform homogenization of the time-harmonic
Maxwell equations in order to determine the effective dielectric
permittivity and effective electric conductivity. We prove that both
these effective parameters depend on pulsation; this phenomenon is known
as the frequency dispersion effect. Moreover, the macroscopic Maxwell
equations also depend on pulsation; they are different for small and
large values of pulsation. |
|
S. Shmarev (Oviedo, Spain)
Localization and blow-up
of solutions to evolution PDEs with nonstandard growth conditions |
| |
|
G. Vallet (Pau, France)
On the stochastic ∆p
problem |
|
In this talk, we will be interested in a result of existence of a
solution to a p-Laplace problem with a stochastic force, when p is a
function of variables t and x. |
|
J.L. Vázquez (Madrid, Spain)
Scaling methods, energies
and entropies in nonlinear diffusion |
|
The long time behavior of nonlinear evolution equations is a classical
topic of the analysis of PDEs. A number of methods have allowed
to make progress in the description of the asymptotic behaviour
of the solutions for equations of parabolic type. I will present three
methods and mention some recent progress. |
|
L. Veron (Tours, France)
Initial trace of positive
solutions of weakly superlinear parabolic equations |
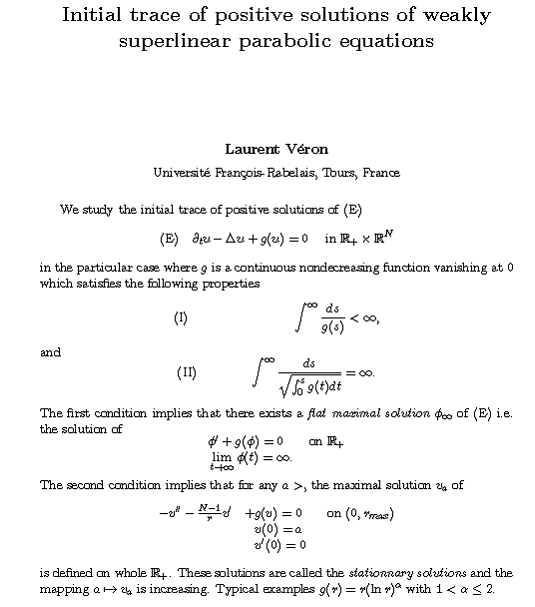 |
| |
| |