Full paper in PDF format:
$%E.-Y. Park and I. Blake, Construction of extended Steiner systems
for information retrieval, Rev. Mat. Complut. 21 (2008), no. 1, 179–190.%$
Construction of Extended Steiner Systems
for Information Retrieval
Eun-Young PARK and Ian BLAKE
Received:
March 2, 2007
Accepted: August 30, 2007
A multiset batch code is a variation of information retrieval where a  -multiset
of items can be retrieved by reading at most one bit from each server. We
study a problem at the other end of the spectrum, namely that of retrieving a
-multiset
of items can be retrieved by reading at most one bit from each server. We
study a problem at the other end of the spectrum, namely that of retrieving a
 -multiset of items by accessing exactly one server. Our solution to the problem
is a combinatorial notion called an extended Steiner system, which was first
studied by Johnson and Mendelsohn (1972). An extended Steiner system
-multiset of items by accessing exactly one server. Our solution to the problem
is a combinatorial notion called an extended Steiner system, which was first
studied by Johnson and Mendelsohn (1972). An extended Steiner system
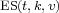 is a collection of
is a collection of  -multisets (thus, allowing repetition of elements
in a block) of a
-multisets (thus, allowing repetition of elements
in a block) of a  -set such that every
-set such that every  -multiset belongs to exactly one block.
An extended triple system, with
-multiset belongs to exactly one block.
An extended triple system, with  and
and 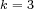 , has been investigated
and constructed previously ["On the existence of extended triple systems" (Bennett and Mendelssohn, 1978), "Extended triple systems" (Johnson and Mendelssohn, 1972)]. We study extended systems
over
, has been investigated
and constructed previously ["On the existence of extended triple systems" (Bennett and Mendelssohn, 1978), "Extended triple systems" (Johnson and Mendelssohn, 1972)]. We study extended systems
over  elements with
elements with 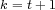 , denoted as
, denoted as  . We show
constructions of
. We show
constructions of 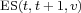 for all
for all  and
and 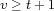 .
.
Key words: information retrieval, batch codes, combinatorial designs, Steiner systems.
2000 Mathematics Subject Classification: 05B05, 05E05, 62K10.
 -multiset
of items can be retrieved by reading at most one bit from each server. We
study a problem at the other end of the spectrum, namely that of retrieving a
-multiset
of items can be retrieved by reading at most one bit from each server. We
study a problem at the other end of the spectrum, namely that of retrieving a
 -multiset of items by accessing exactly one server. Our solution to the problem
is a combinatorial notion called an extended Steiner system, which was first
studied by Johnson and Mendelsohn (1972). An extended Steiner system
-multiset of items by accessing exactly one server. Our solution to the problem
is a combinatorial notion called an extended Steiner system, which was first
studied by Johnson and Mendelsohn (1972). An extended Steiner system
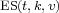 is a collection of
is a collection of  -multisets (thus, allowing repetition of elements
in a block) of a
-multisets (thus, allowing repetition of elements
in a block) of a  -set such that every
-set such that every  -multiset belongs to exactly one block.
An extended triple system, with
-multiset belongs to exactly one block.
An extended triple system, with  and
and 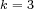 , has been investigated
and constructed previously ["On the existence of extended triple systems" (Bennett and Mendelssohn, 1978), "Extended triple systems" (Johnson and Mendelssohn, 1972)]. We study extended systems
over
, has been investigated
and constructed previously ["On the existence of extended triple systems" (Bennett and Mendelssohn, 1978), "Extended triple systems" (Johnson and Mendelssohn, 1972)]. We study extended systems
over  elements with
elements with 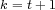 , denoted as
, denoted as  . We show
constructions of
. We show
constructions of 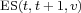 for all
for all  and
and 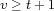 .
.