 -parameter symmetry
group of a hypersurface
-parameter symmetry
group of a hypersurface 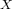 in projective space was equivalent to failure of
versality of a certain unfolding. Here we study in detail (reduced) plane curves
of degree
in projective space was equivalent to failure of
versality of a certain unfolding. Here we study in detail (reduced) plane curves
of degree 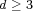 , excluding the trivial case of cones.
, excluding the trivial case of cones.
Full paper in PDF:
$%A. A. du Plessis and C. T. C. Wall, Curves in P2(C) with 1-dimensional symmetry,
Rev. Mat. Complut. 12 (1999), no. 1, 117–132. %$
Matematisk Institut ny Munkegade Aarhus Universitet 8000 Aarhus C. — Denmark
matad@mi.aau.dk | Department of Pure Mathematics The University of Liverpool Liverpool I. 69 3BX — England
C.T.C.Wall@liverpool.ac.uk |
ABSTRACT
In a previous paper we showed that the existence of a  -parameter symmetry
group of a hypersurface
-parameter symmetry
group of a hypersurface 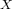 in projective space was equivalent to failure of
versality of a certain unfolding. Here we study in detail (reduced) plane curves
of degree
in projective space was equivalent to failure of
versality of a certain unfolding. Here we study in detail (reduced) plane curves
of degree 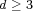 , excluding the trivial case of cones.
, excluding the trivial case of cones.
We enumerate all possible group actions — these have to be either semisimple
or unipotent — for any degree  . A
. A  -parameter group can only occur if
-parameter group can only occur if
 . Explicit lists of singularities of the corresponding curves are given in
the cases
. Explicit lists of singularities of the corresponding curves are given in
the cases 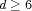 . We also show that the projective classification of these curves
coincides — except in the case of the group action with weights
. We also show that the projective classification of these curves
coincides — except in the case of the group action with weights ![[-1,0,1]](vol12-1g11x.png) —
with the classification of the singular points.
—
with the classification of the singular points.
The sum  of the Tjurina numbers of the singular points is either
of the Tjurina numbers of the singular points is either 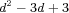 or
or 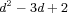 while, for
while, for 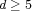 , if there is no group action we have
, if there is no group action we have
 . We give
. We give  in the semi-simple case; in the unipotent
case, we determine the values of both
in the semi-simple case; in the unipotent
case, we determine the values of both  and
and  .
.
In the semi-simple case, we show that the unfolding mentioned above is also
topologically versal if 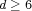 ; in the unipotent case this holds at least if
; in the unipotent case this holds at least if  .
.
1991 Mathematics Subject Classification: 14B05, 14NO5.