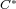Full paper in PDF format:
$%M. Loaiza and H. Upmeier, Toeplitz C*-algebras on super-Cartan domains, Rev. Mat. Complut. 21 (2008), no. 2, 489–518.%$

We study Hilbert spaces of super-holomorphic functions (including anti-commuting Grassmann variables) in the setting of bounded symmetric domains, more precisely for the matrix ball of arbitrary size. Our main results concern the classification of irreducible representations of the associated Toeplitz 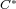-algebra and an explicit decomposition of the super-Bergman space as a direct sum of vector-valued (ordinary) Bergman spaces.

Key words: Toeplitz operator algebras, Grassmann variables, supersymmetry, Berezin quantization, Cartan domains, vector-valued Bergman spaces.
2000 Mathematics Subject Classification: 47L80, 32M15.