Full paper in PDF format:
$%D. D. Haroske and L. Skrzypczak, Entropy and approximation numbers of embeddings of function spaces
with Muckenhoupt weights, I, Rev. Mat. Complut. 21 (2008), no. 1, 135–177.%$
Entropy and Approximation Numbers
of Embeddings of Function Spaces
with Muckenhoupt Weights, I
Dorothee D. HAROSKE and Leszek SKRZYPCZAK
| | Faculty of Mathematics & Computer Science
Adam Mickiewicz University
Ul. Umultowska 87
61-614 Poznań — Poland
lskrzyp@amu.edu.pl |
| |
Received: July 10, 2007
Accepted: August 30, 2007
We study compact embeddings for weighted spaces of Besov and Triebel-Lizorkin
type where the weight belongs to some Muckenhoupt 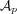 class. For weights
of purely polynomial growth, both near some singular point and at infinity, we
obtain sharp asymptotic estimates for the entropy numbers and approximation
numbers of this embedding. The main tool is a discretization in terms of wavelet
bases.
class. For weights
of purely polynomial growth, both near some singular point and at infinity, we
obtain sharp asymptotic estimates for the entropy numbers and approximation
numbers of this embedding. The main tool is a discretization in terms of wavelet
bases.
Key words: wavelet bases, Muckenhoupt weighted function spaces, compact
embeddings, entropy numbers, approximation numbers.
2000 Mathematics Subject Classification: 46E35, 42C40, 42B35, 41A46, 47B06.
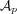 class. For weights
of purely polynomial growth, both near some singular point and at infinity, we
obtain sharp asymptotic estimates for the entropy numbers and approximation
numbers of this embedding. The main tool is a discretization in terms of wavelet
bases.
class. For weights
of purely polynomial growth, both near some singular point and at infinity, we
obtain sharp asymptotic estimates for the entropy numbers and approximation
numbers of this embedding. The main tool is a discretization in terms of wavelet
bases.