N. Alikakos (University of Athens, Greece)

A maximum principle for systems with variational structure and an application to standing waves

We establish via variational methods the existence of a standing wave together with an estimate on the convergence to its asymptotic states for a bistable system of partial differential equations on a periodic domain. The main tool is a replacement lemma which has as a corollary a maximum principle for minimizers.

H. Attar (Siemens, Germany)

Simulation of Elastoplastic Deformation of Steel in Rolling Process

In the production lines of hot strip rolling of steel, there is still a considerable amount of energy and material wasted due to difficulties with the optimal shape-formation of the slabs. In this project we have intended to derive and implement a model for simulation of (elasto)plastic deformation of the steel plates during the rolling process. The results of such simulation are very useful in proper control of the rolling mills throughout the process. Our model is based on finite strain elastoplasticity with J2-flow plasticity, and frictional contact between rolls and the slab. A brief overview of the underlying theories and selected results are presented.

G. Bellettini (Sapienza Università di Rome, Italy)

Upper estimates of the area of graphs of discontinuous maps fromthe plane to the plane

We discuss the issue of computing the area of the graph of a discontinuous map from the plane to the plane.

This turns out to be the problem of approximating a two-codimensional nonsmooth surface in four dimensional space, with a sequence of smooth surfaces, keeping the approximating areas as low as possible.

We will focus only on an upper bound, and for a class of discontinuous maps with well behaved jump and traces.

C. Budd (University of Bath, UK)

Mathematical models of rock folding

In this talk I will discuss a number of different mathematical models for the folding of rock. These are all formulated as either fourth order PDEs with constraints or as constrained second order variational problems. We solve these with a mixture of methods.

I will show how each of these models describes rock folding patterns observed in nature which include singular types of behaviour such as chevron patterns and kink bands.

This is joint work with the Bath ESRs Leire Gorrochategui Gregorio and Amine Chakhchoukh.

 

L. T. T. Bui (Zurich, Switzerland)

Some results on the forward-backward equations

(joint work with Flavia Smarrazzo and Alberto Tesei).

We study the forward-backward problems which arise in many applications in physical and biological phenomena. Since the forward-backward problems are in general ill-posed, we investiagte the irregularized problems which are expected to be well-posed. By the different physical meaning of the regularized terms, we obtain the different solutions of the original problems. In our work, we address the Sobolev regularization and Cahn-Hilliard regularization.

E. Campillo (Erlangen, Germany)

Modelling and Analysis of Electrowetting and other Electrokinetic Phenomena

M. Chipot (University of Zurich)

Existence results for some Functional Elliptic Equations

A. N. Dao (Universidad Complutense de Madrid)

Quenching phenomenon of singular parabolic problems with L¹ initial data

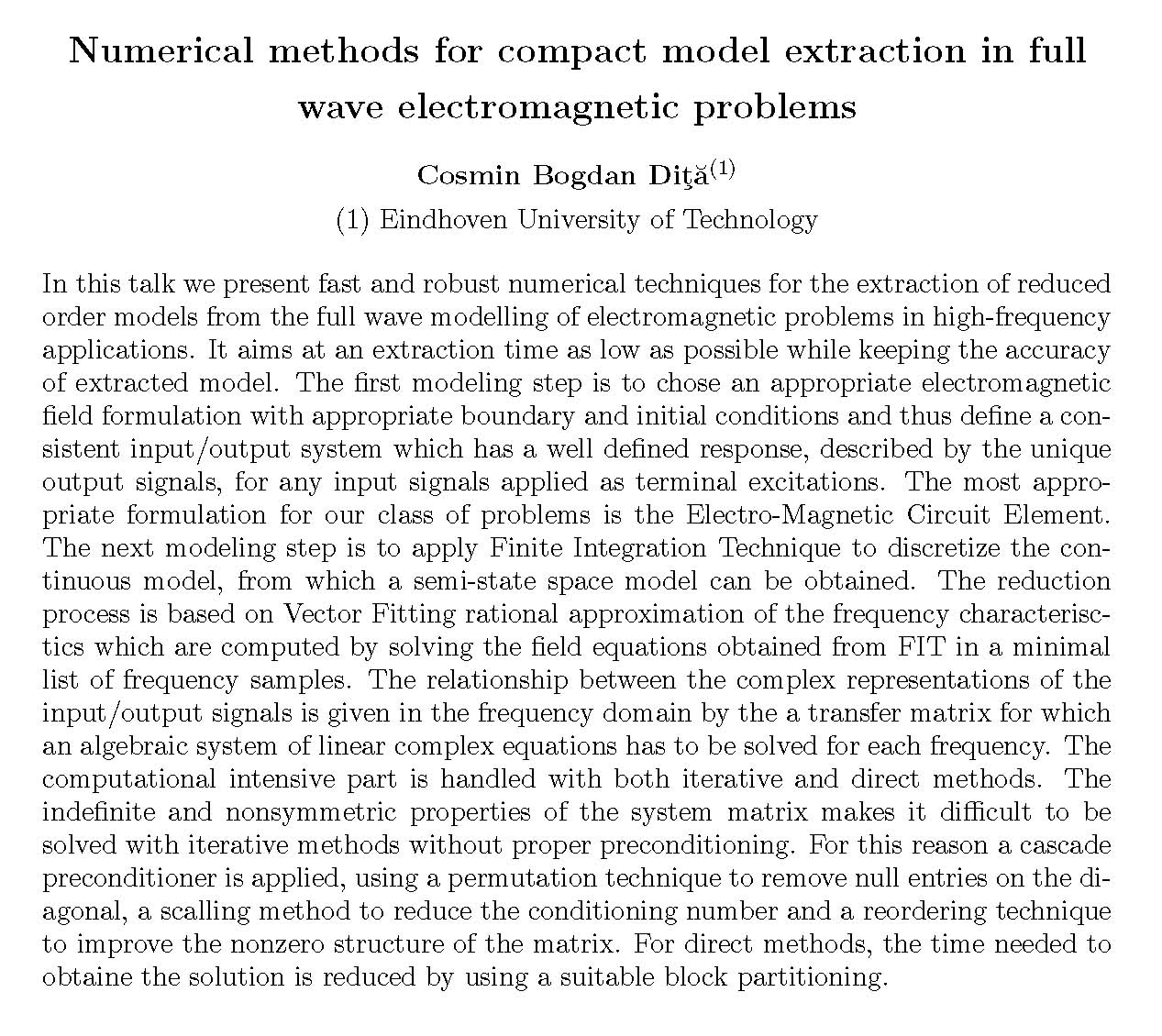
C. B. Dita (Eindhoven, The Neherlands)

Numerical methods for compact model extraction in full wave electromagnetic problems

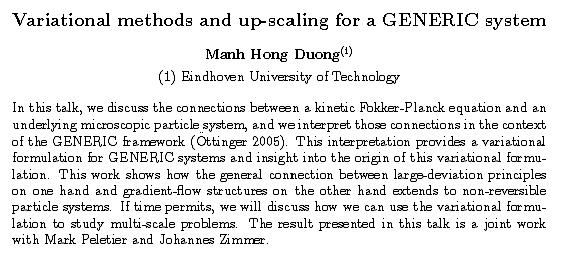
M. H. Duong (Eindhoven, The Neherlands)

Variational methods and up-scaling for a GENERIC system

R. I. Fábregas (Rome, Italy)

A three-dimensional model for the initial progress of caries

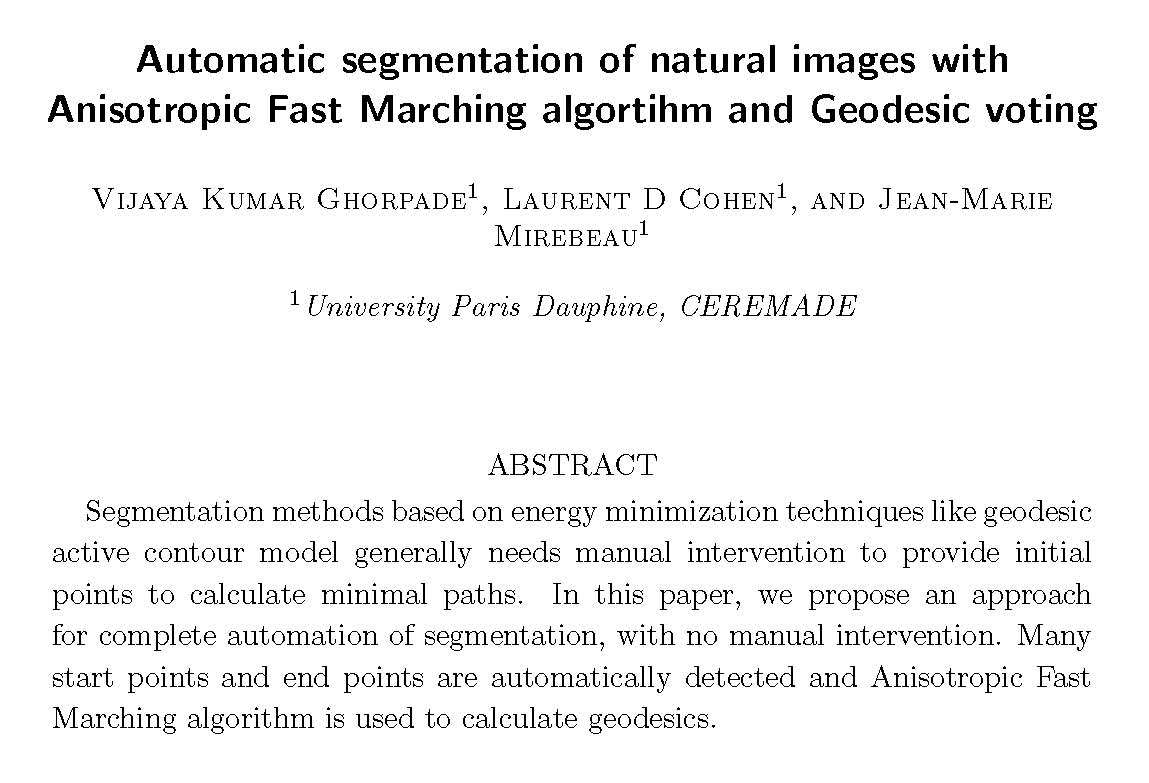
V. K. Ghorpade (Paris, France)

Automatic segmentation of natural images with Anisotropic Fast Marching algorithm and Geodesic voting

A. Ghosh (Paris, France)

On the Cuspless Sub-Riemannian Geodesics in R³×S²

We studied the cuspless curves in three dimensional Euclidean space that minimize the energy functional ∫√(κ² + β²)ds (with integral limits 0 to L) defined for each spatial arc-length parameterized curve with curvature κ and length (free) L ≥ 0. To compute the stationary curves, we took the Lagrangian approach used by Bryant and Grifiths for similar minimization problems. We have also shown that two other approaches, a direct variational method in R3 and the Hamiltonian approach using the Pontryagin’s Maximum Principle (PMP) give the same ODE’s for the stationary curves (that are locally minimizing due to PMP). We show that using the spatial arc-length parameterizations is advantageous in our case over the sub-Riemannian arc-length parameterization. The Bryant and Griffiths approach leads us to explicit formulas for the stationary curves. The formulas allow us to extract geometrical properties of the cuspless sub-Riemannian geodesics, such as planarity conditions and explicit bounds on the torsion. Moreover, they allow a study of the symmetries of the associated exponential map, and they allow to numerically solve both the initial and the boundary value problem, and to numerically compute the range of the exponential map. As a result, we characterize necessary conditions on the boundary conditions for which our optimization is well-posed.

G. Grün (Friedrich-Alexander-Universität Erlangen-Nürnberg, Germany)

On finite speed of propagation of solutions to the stochastic porous-media equation

A new method -- based on energy estimates -- is presented to establish finite speed of propagation for the stochastic porous-media equation with multiplicative noise. The technique is rather general and may be adapted to further propagation phenomena or to stochastic versions of other degenerate parabolic equations than the porous-media equation. It is based on a joint work with Julian Fischer.

M. A. Herrero (Universidad Complutense de Madrid, Spain)

Mathematical problems in radiotherapy

Radiotherapy, the use of ionizing radiation to destroy malignancies, is a common treatment for many solid tumours . In spite of its widespread clinical use, a number of uncertainties remain concerning basic aspects , as for instance i) the best possible choice of a prescription dose, and ii) the manner in which actual dosimetries , close enough to optimal ones, could be delivered on target. In this lecture a description of the modelling problems related to the previous issues will be provided, and some mathematical results for such problems will be presented.

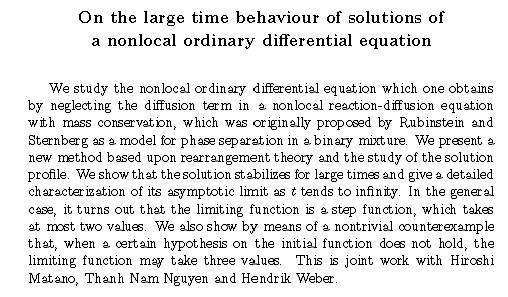
D. Hilhorst (Université de Paris-Sud XI, France)

On the large time behaviour of solutions of a nonlocal ordinary differential equation

R. Kimmel (Technion - Israel Institute of Technology)

Computational Metric Geometry

Treating shapes as metric spaces allows us to apply modern metric geometry tools to match, process, represent, and find the discrepancy between various objects. Hearts, faces, hands, and the cortical surface of the brain are just a few examples of such geometric structures for which an accurate comparison tool is vital. The questions we will try to answer in this talk are:

1. What are the invariants one should apply when analyzing such manifolds?

2. What are the most efficient computational tools we can utilize to compute these invariants?

R. Knabner (Friedrich-Alexander-Universität Erlangen-Nürnberg, Germany)

Towards a Rigorous Mathematical Modelling of Colloid and Colloidal-Influenced Transport in Porous Media

Groundwater and soil pollution has become a major threat in the last century. The risk assessment by means of numerical simulations of the long term persistance and spreading of contaminants in the subsoil has to take into consideration a variety of hydrophysicochemical processes. In this context, the modelling of colloid dynamics plays a decisive role, since contaminant transport is highly influenced by the movement and distribution of colloidal particles, which furthermore may itself act as contaminants. This talk deals with the rigorous mathematical modelling of colloid and colloidal-influenced transport in porous media, in particular, taking into account the effects of electrostatic particle–particle and particle–surface interaction, attachment and detachment processes on the surface of the pore matrix, flocculation, and the induced influence on the solvent movement. The presented effective models are either derived by rigorous or formal averaging procedures by periodic homogenization. The effective coefficients possess closed form expressions, which contain the information of the underlying pore geometry. All models are implemented using mass-conservative mixed FE methods that are fully implicit in time.

H. Koçak (Bath University)

Blow-up in Third-order PDEs

We discuss blow-up and global behaviour of the third order dispersion equations via their reduced second-order ODE form. Numerical and analytical results are discussed and the profiles are displayed.

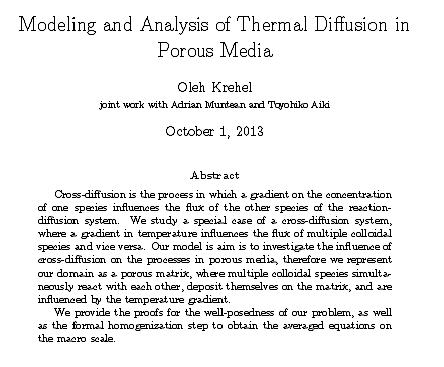
O. Krehel (Eindhoven, The Neherlands)

Modeling and Analysis of Thermal Diffusion in Porous Media

P. Kyriazopoulos (Paris, France)

A pattern formation mechanism for species coexistence

Organisms and ecosystems tend to form spatial patterns even in uniform environments. A variety of ecological and biological systems have been successfully modelled by reaction-diffusion equations which generate stationary spatial patterns through diffusion-driven instability mechanisms [3]. However, very few model studies have addressed the impact that pattern formation may have on the spatial distribution of interacting species and how this affects coexistence and biodiversity.

We shall discuss a scenario of species coexistence associated with bistability of uniform and patterned states of two competing plant species in water limited ecosystems. These species are related to one another by a tradeo between investment in growing taller shoots and investment in increasing root-to-shoot ratio. The interaction between the two species is studied along a rainfall gradient using a modified version of the vegetation model introduced by Gilad et al. [1] that includes interspecific competition for light. Simulations and numerical bifurcation analysis of the model indicate that spatially localized structures can be formed in a subregion of the bistability precipitation range. The localized structures consist of confined domains of the patterned state in a background of the uniform state. This suggests a spatial coexistence mechanism based on a generic mathematical property of bistable pattern-forming systems which may be applicable to many other contexts of ecological communities [2].

References
[1] E. Gilad, M. Shachak, and E. Meron. Dynamics and spatial organization of plant communities in water-limited systems. Theoretical Population Biology, 72:214-230, 2007.
[2] E Knobloch. Spatially localized structures in dissipative systems: open problems. Nonlinearity, 21(4):T45, 2008.
[3] A.M. Turing. The chemical basis of morphogenesis. Philosophical Transactions of the Royal Society of London, series B, 237:37-72, 1952.

J. M. Mazón (Universidad Complutense de Madrid, Spain)

An optimal matching problem for Euclidean distance

T. Mingazzini (Zurich, Switzerland)

Global Null Controllability of the One-Dimensional Nonlinear Slow Diffusion Equation

J. M. Morel (Université de Paris-Sud XI, France)

A PDE formalization of Retinex theory explaining the human color perception

(Joint work with Ana Belen Petro and Catalina Sbert)

In 1964 Edwin H. Land formulated the Retinex theory, the first attempt to simulate and explain how the human visual system perceives color. This theory was further formalized into an algorithm by Land and McCann in 1971. Unfortunately, the Land-McCann original algorithm is both complex and not fully specified. It computes at each pixel an average of a very large set of paths on the image. I shall prove that if the paths are assumed to be symmetric random walks, the Retinex solutions satisfy a discrete Poisson equation. This formalization yields an exact and fast implementation using only two FFT’s. Several experiments on color images illustrate the effectiveness of the Retinex theory. The algorithm can be tried online at www.ipol.im in the "color and contrast" section.

M. A. Peletier (Technische Universiteit Eindhoven, The Netherlands)

Exact crystallization in a block copolymer model

One of the holy grails of material science is a complete characterization of ground states of material energies. Some materials have periodic ground states, others have quasi-periodic states, and yet others form amorphic, random structures. Knowing this structure is essential to determine the macroscopic material properties of the material. In theory the energy contains all the information needed to determine the structure of ground states, but in practice it is extremely hard to extract this information.

In this talk I will describe a model for which we recently managed to characterize the ground state in a very complete way. The energy describes the behaviour of diblock copolymers, polymers that consist of two parts that repel each other. At low temperature such polymers organize themselves in complex microstructures at microscopic scales.

We concentrate on a regime in which the two parts are of strongly different sizes. In this regime we can completely characterize ground states, and even show stability of the ground state to small energy perturbations.

This is work with David Bourne and Florian Theil.

M. A. Pozio (Sapienza Università di Rome, Italy)

Qualitative behavior of solutions of doubly nonlinear parabolic equations with a linear reaction term

We study the qualitative properties of explicit self--similar solutions for the doubly nonlinear diffusion equation with a positive linear reaction term. For this problem u=0 is an unstable stationary solution and we prove that its unstable manifold has infinite Hausdorff dimension, even if the spatial domain is bounded, provided that the degree of homogeneity of the diffusion operator is superlinear. Indeed explicit self--similar solutions exist that vanish in the limit for t going to infinity while their support shrinks to an arbitrarily chosen point or a ball, a line, an hyperplane, an infinite cylinder and so on. These results are in marked contrast with the case of non-degenerate semilinear equations and had already been proved by H. Matano and the author, in the porous medium case with a reaction term.

A. M. Ramos (Universidad Complutense de Madrid, Spain)

Mathematical modelling of Lithium-ion batteries

In this talk we will discuss the mathematical modelling of the performance of Lithium-ion batteries. A mathematical model based on a macro-homogeneous approach developed by John Neuman will be presented.

J. Rubinstein (Technion - Israel Institute of Technology)

Hydroelastic waves in the cochlea and the place principle

We present a model for the hydro-elastic waves in the cochlea, and an analysis of it. In particular we show how to reduce the problem to a one-dimensional Schrodinger equation. Using the reduced model we derive the precise functional form of the place principle. Finally we mention clinical application of the project.

T. Savitska (Rome, Italy)

On the asymptotic behaviour of some nonlocal parabolic problem associated with p-Laplace operator

E. Schiavi (Universidad Rey Juan Carlos de Madrid, Spain)

Nonlinear problems in Magnetic Resonance Image Denoising and Superresolution

Medical image processing is a growing field in medicine and mathematics which aims to improve the diagnostic power of some acquisition data modalities such as MRI, fMRI, PET, MEG, CT, etc.

The existing trade off between high image quality and reduced patient scanning time generates low Signal to Noise (SNR) images and this introduces the need for accurate MRI processing and restoration.

In this talk we shall consider some recent image processing related mathematical problems with a particular view to image denoising and superresolution.

Our research is based at Fundación CIEN-Fundación Reina Sofía, Madrid, Spain where a interdisciplinary group of scientists is working on biomarkers for neurological deseases such as Alzheimer and Parkinson.

S. O. Tamasoiu (Paris, France)

Stabilization and Boundary Control for Balance and Conservation Laws

A. Tesei (Sapienza Università di Rome, Italy)

Pseudo-parabolic regularization of forward-backward parabolic equations

(joint work with M. Bertsch and F. Smarrazzo)

It is known that solutions of initial boundary value problems for regularized forward-backward equations of Perona-Malik type can develop singularities. This motivates studying these problems in spaces of Radon measures. We shall discuss existence and qualitative properties of solutions of this kind obtained by a suitable approximation procedure. The results have been presented in joined papers with M. Bertsch and F. Smarrazzo.

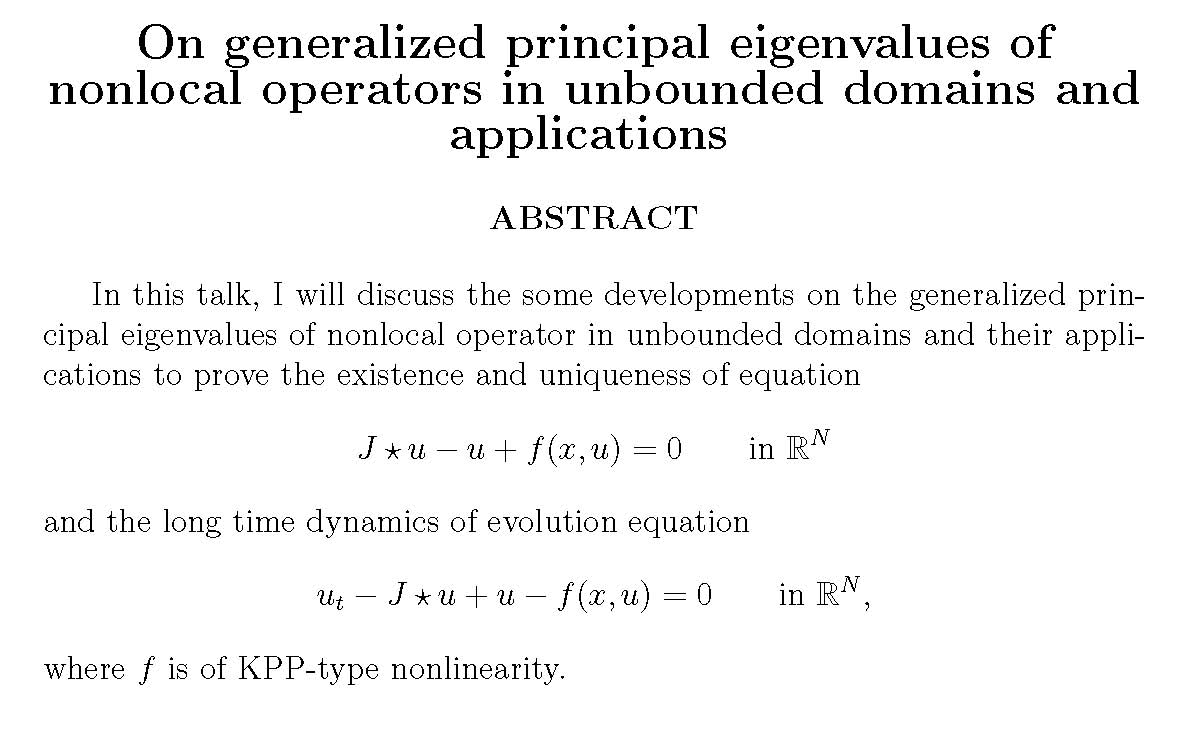
H. Vo (Eindhoven, The Neherlands)

On generalized principal eigenvalues of nonlocal operators in unbounded domains and applications

H. C. Vu Do (Orsay, France)

A generalised finite volume method for density driven flows in porous media

We apply a semi-implicit scheme in time together with a generalized finite volume method for the numerical solution of density driven flows in porous media; it comes to solve a nonlinear convection-diffusion parabolic equation for solute transport together with an elliptic equation for the pressure. We compute the solutions for three specific problems : a problem involving a rotating interface between salt and fresh water, the Henry problem in space dimension two, and a three dimensional salt pool problem. We use adaptive meshes, based upon square volume elements in space dimension two and cubic volume elements in space dimension three.

J. Zimmer (University of Bath, UK)

Entropic flows, stochastic perturbations and microscopic models

As starting point, different ways to link some PDEs of diffusive type to particle models will be reviewed. Typically, the PDE will be related to a minimisation problem of a large deviation rate function from probability. Here various approaches are possible and will be sketched. The underlying microscopic process contains more information, notably fluctuations around the minimum state described by the deterministic PDE. Can stochastic terms be derived which model this additional information, in a way that is compatible to the limit passage via large deviations (and the geometric structure, such as the Wasserstein setting)? This question will be investigated for the simplest possible case of linear diffusion.